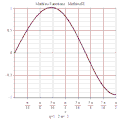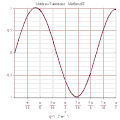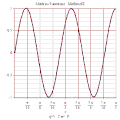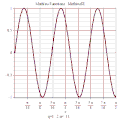
马丢函数
马丟函数(法語:)是1868年法國數學家以米里迂·拉·馬丢因研究数学物理所推得的特殊函數,下列马丟方程的解析解:
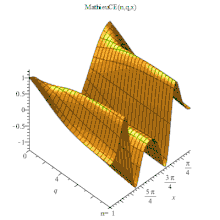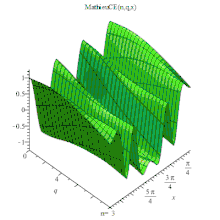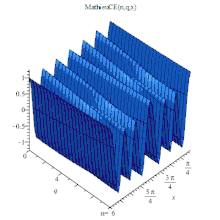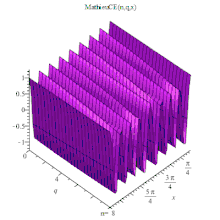
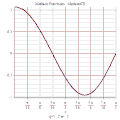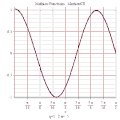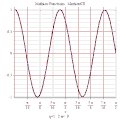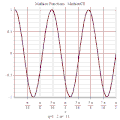
MathieuCE 3D
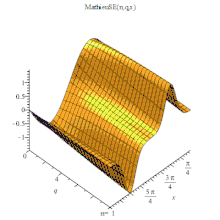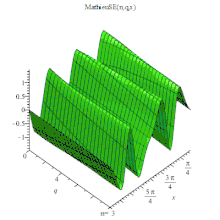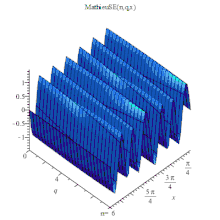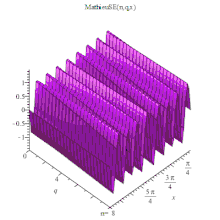
MathieuSE 3D
马丟方程有两个线性无关的解:
- 奇数解
MathieuCE(n, q, x),或记为,
- 偶数解
MathieuSE(n, q, x).或记为 称为基本解[1]
周期性
马丟函数 MathieuC(a,q,z) 或 MathieuS(a,q,z) 只有一个是周期为 或的周期解,另一个不是。
马丟函数 MathieuC(a,q,z) 和 MathieuS(a,q,z) 两者都有是周期为(n≥2)的周期函数。 [1]
正交性
特征方程
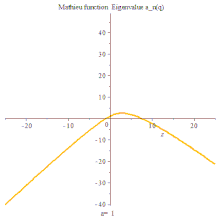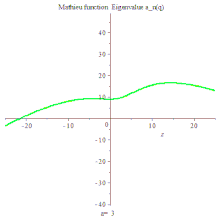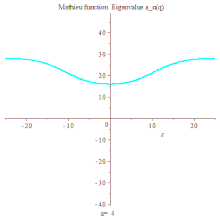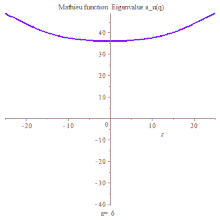
Mathieu Eigen value a(n,q)

Mathieu eigenvalue b(n,q)
马丟方程的特征方程是[1]
对于给定的v,q, 上列特征方程给出无穷多个a、b解称为特征值。
特例
夫洛开解
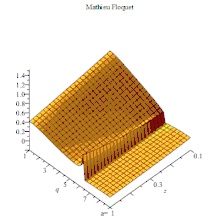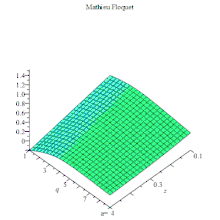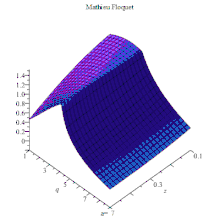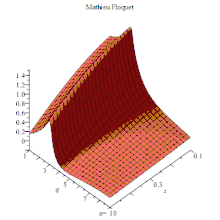
Mathieu Floquet
马丟函数中,如果 是一个周期为的解,并满足下列条件
,其中与x 无关,则此解称为夫洛开解。
- 级数展开
参考文献
- 王竹溪 郭敦仁 603
- Frank p659
- Frank p660
- 王竹溪 郭敦仁 《特殊函数概论》 第十二章 马丟函数 北京大学出版社 2000
- Frank J Oliver NIST Handbook of Mathematical Functions,Cambridge University PRESS, 2010
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.