شيفي
في الهندسة، الشيڤي (بالإنجليزية: Cevian) أو قاطع المثلث هو خطٌ يمر برأس مثلث، ويقطع الجانب المقابل لذلك الرأس.[1][2] تُعدُّ المتوسطات ومنصفات الزوايا من الشيڤيّات. يُسمى الشيڤي نسبةً إلى عالم الرياضيات الإيطالي جيوفاني شيفا، الذي أثبت مبرهنة معروفة عن الشيڤيات والتي تحمل اسمه أيضًا.[3]
الطول
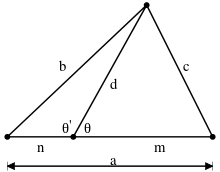
مثلث بطول سيفيان د
نظرية ستيوارت
يمكن تحديد طول قاطع المثلث من خلال مبرهنة ستيوارت: في الرسم الآتي ، يُحسب طول الشيڤي d عبر الصيغة:
زاوية منصف
إذا كان القاطع منصف زاوية ، فإن طوله يخضع للصيغة
و [4]
و
حيث مقياس نصف القطر s = (a+b+c)/2 .
ضلع الطول a مقسوم بالنسبة b:c .
انظر أيضاً
- هندسة نقطة الكتلة
- نظرية مينيلوس
ملحوظات
- Coxeter، H. S. M.؛ Greitzer، S. L. (1967). Geometry Revisited. Washington, DC: اتحاد الرياضيات الأمريكي. ص. 4. ISBN:0-883-85619-0.
- Some authors exclude the other two sides of the triangle, see Eves (1963)
- Lightner، James E. (1975). "A new look at the 'centers' of a triangle". مجلس معلمي الرياضيات الوطني. ج. 68 ع. 7: 612–615. JSTOR:27960289.
- Johnson, Roger A., Advanced Euclidean Geometry, Dover Publ., 2007 (orig. 1929), p. 70.
مراجع
- Eves، Howard (1963)، A Survey of Geometry (Vol. One)، Allyn and Bacon
- Ross Honsberger (1995). Episodes in Nineteenth and Twentieth Century Euclidean Geometry, pages 13 and 137. Mathematical Association of America.
- Vladimir Karapetoff (1929). "Some properties of correlative vertex lines in a plane triangle." American Mathematical Monthly 36: 476–479.
- Indika Shameera Amarasinghe (2011). “A New Theorem on any Right-angled Cevian Triangle.” Journal of the World Federation of National Mathematics Competitions, Vol 24 (02), pp. 29–37.
- بوابة رياضيات
- بوابة هندسة رياضية
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.